Second Scientific Lecture-Course: Warmth Course

Lecture XII

Stuttgart, March 12th, 1920.

My dear friends,

The experiments we had anticipated carrying out today we will unfortunately have to postpone until tomorrow. At that time they will be arranged so as to show you what is necessary if I am to prove to you all that I wish to prove. Today, therefore, we will consider some things which, together with the experiments of tomorrow, will enable us to bring our observations to a conclusion the following day.

As a help toward the understanding of the being of heat, I wish to call your attention to a certain fact. This fact is one which we must take into account in developing our ideas on this subject, and it is that there is a certain difficulty in understanding what is really involved in a transparent body. I am not now speaking of transparency in connection with heat. You will see, however, when we have finished that we can get helpful ideas for understanding heat from the realm of light.

I said there was a certain difficulty in understanding what a relatively transparent body is and what an opaque body is as these reveal themselves under the influence of light. I have to express myself in a different way from that ordinarily used. The ordinary method of expression in physics would be as follows: an opaque body is one that by some peculiar property of its surface reflects the rays of light that fall on it and thus become a visible body. I cannot use this form of expression because it is not a reflection of the facts, it is a statement of a preconceived theory and is not by any means to be taken as self-evident.

For to speak of rays, of light rays, is theoretical. I have dealt with that in my former course. What we meet in reality is not light rays, but an image and it is this we must hold firmly in mind. As a matter of fact, we cannot simply say: a transparent body is one that by virtue of its inner molecular properties passes light through, and an opaque body is one that throws the light back. For how can such a theory be substantiated? Recollect what I have said to you about the relations of the various realms of reality. We have solids, fluids, gaseous bodies, heat, X, Y, Z and below the solid and bordering on it the U region, and you can see that the light realm must have a relation to heat and so also must the realm of chemical activity. On the other side that which we meet, so to speak, as the fluid nature in heat or in gases must have a relation to the essence of tone. For tone appears alone with the occurrence of condensation and rarefaction in gases or aeriform bodies. We may therefore suspect that where we have assumed X, Y, Z, we will find the essence of light. Now the question is whether we have to look for the explanation of transparency of certain bodies is not to be immediately derived from the nature of light, nor from the relation of light to these bodies.

We have the U region and this U region must have a relation to the solids on the surface of the earth. We must first ask the question and seek to apply the answer to this question to our consideration of these things. What influence has the U region on solids and can we from the nature of this influence derive anything that will show use the difference between transparent bodies and the ordinary non-transparent metals? This question must be considered and the answer to it will appear when we extend further our ideas of yesterday in regard to heat by the addition of certain other conceptions.

Note now, the warmth phenomena naturally are considered as belonging to the realm of physics. Such things as conduction have been included, thought of in the way I have described to you. This spreading of heat through conduction or flow of the heat condition either through a body or from one body to another one touching it has been observed. The flow has been conceived of as though a kind of fluid were involved, and the picture is of a liquid flow. It may be compared to something readily observable in the objective world, namely the water in a brook which is at one point now, and a moment later is at a distant point. Thus is pictured the flow of heat from one spot to another when the so-called conduction of heat takes place. The phenomenon are to be found in Fourrier (other investigators might also be cited.) Let us consider these a little from our own point of view and see if we can establish their validity.

Imagine that we have a body bounded by a definite wall, say of metal (Fig. 1). Assume the wall to extend indefinitely above and below, and suppose it to consist of some sort of metal. Let us place boiling water in contact with the wall on one side holding it at a temperature U₁ which in this case is 100°C. On the other side we place melting ice to hold the wall at a temperature U₂ which in this special case will be 0°C.

Considering the entire phenomenon you will see that we have to do with a difference, here U₁, here U₂; and U₁ and U₂ gives us the temperature difference. Upon this difference depends the fact that we have a conduction of heat. Obviously, this transfer of heat will proceed otherwise when the difference is small, a small quantity of heat is transferred to attain equilibrium, and when the difference is great a larger quantity is transferred. Thus I may say that the quantity of heat needed to attain a certain condition depends on this temperature difference, U₁ - U₂. Furthermore, it will depend not only on the difference U₁ - U₂, but on the thickness of the wall which I may denote by L, becoming greater when this is large and less when it is small. That is, the amount of heat transferred is inversely proportional to L. I may calculate for a given area that I will call Q, how much heat I will need to get a certain degree of conduction. The greater Q is, the greater will be the amount. Thus the amount of heat is directly proportional to Q and I must multiply by this factor.

Finally, the whole process is dependent upon time. A greater effect is produced by permitting a given amount of heat to act for a longer time, a smaller effect in a less time. Therefore I have to multiply by the time. Obviously then, I must multiply through by a constant representing the heat itself, by something involving heat, since none of the quantities so far mentioned include the heat and thus cannot by themselves give the quantity of heat, W, which I wish to secure. This quantity of heat, W, is directly proportional to L. Now if you equate all the other factors with U₁ and U₂, you are expressing what really flows and this not a heat quantity, essentially, nor dependent directly on a heat quantity, but is a temperature fall, a difference in level. Please keep this in mind. Just as when we pour water through a sluice and turn a paddle wheel, and the motion is due to the energy arising from a different in level, so there we have to do with a drop from one level to another, and it is this we must keep our attention on.

Now we have to take up another consideration of Fourrier's to draw nearer to the being of heat. We will work over the ordinary concepts as it were so as to move nearer to reality than the physicists of the 20^th century. So far I have taken into consideration only what pertains to the conducting of heat from one spot to another, but I can assume that something goes on in the body itself. Let me now ask a question. Suppose we assume that the progress of heat instead of being uniform from left to right was non-uniform, then the formula would have to apply to the inner lack of uniformity. If the irregularity in the partition of heat is present I must bring it into my considerations in some way. I must bring in the differences that reveal themselves within, that is, what takes place in the body as the temperature effects equalize themselves. As you can easily see, my formula is applicable to the process. I can say

	U1 - U2
W =	—————	t, c, q.
	L

That represents what takes place here. I will not consider the whole thickness of the wall, but deal with small portions of it, and will consider what happens in these small portions, as over the entire distance it is expressed by the factor

U1 - U2

—————

L

It is thus a question of dealing with minute distances within the body. To do this, I employ the differential ratio du

du

——

dx

where dx represents an infinitesimal movement of heat. If this is considered for an instant of time, I must multiply by dt, this being left out of account if I do not consider the time. Thus we have W as an expression of the quantity of heat transferred through small distance in order to equalize the temperature within the body. The following formula expresses the effects of temperature fall within the body:

	du
W =	——	dt, c, q.
	dx

In relation to this, I will ask you please to consider what we took up yesterday in a sketchy way, which will be clearer tomorrow when we have carried out the necessary experiments. Today, I will simply mention it, since we must keep it in mind. I refer to the relation between heat, light and chemical effect in the spectrum. Yesterday, your attention was called to the following fact: when we have an ordinary terrestrial spectrum, in the middle is the light effect proper, towards one end (Fig. 2, arrow) heat effects, toward the other end the chemical effects Now we have to consider the following.

We have seen that when we construct a picture of this spectrum, we must not think of light, heat and chemical effects as stretched out in a straight line. We go toward the left to approach the warm end of the spectrum and toward the right to approach the chemically active end. (Fig. 2) thus, it is not possible to remain in the lane of the pure light effects if we wish to symbolize the heat effects; nor can we remain in this place if we wish to symbolize the chemical effects. We have to move out of this plane.

Now to visualize the whole matter, let us make clear to ourselves how we must really represent a heat quantity working within a body by means of our formula. How must we represent qualitatively the relation between it and the chemical effect? We will not do this properly until we take into account the fact that we go one way to reach the heat and the opposite way to reach the chemical effects. This fact must be kept in mind if we would orient ourselves. So when we consider W as a positive quantity here (or we might consider it negative) then we have to consider the corresponding chemical effect as:

	du
W = -	——	dt, c, q.  (1)
	dx

The foregoing equation corresponds to the chemical effect, and this one:

	du
W = +	——	dt, c, q.  (2)
	dx

corresponds to the heat effect.

As a matter of fact, these things demonstrate for us an important point. This point is that when we use formulae we cannot handle the mathematical quantities merely as such if we at the same time expect the formulae to express the relations within a field of actual effects, an observed realm, where heat and chemical action are manifesting themselves. In ordinary combustion, for instance, where we wish to bring heat and chemical effects into relation, we must, if we use formulae, set down as positive what represents heat and as negative what represents chemical effect.

Now if you carry your considerations further, you may make the following statement: When we think of heat as extending in one direction, so to speak, and chemical action as extending in the opposite, then we have what is essential in light left in a plane at right angles to the imagined chemical action-heat lines and between them. But if you have reserved positivity for heat and negativity for chemical action, you cannot use either of these for light effects. At this point you have to apply to the light effects a set of facts which today are only vaguely felt and not by any means explained, namely the relation between positive and negative numbers and imaginary numbers. When you are dealing with light phenomena you have to say:

	du
W = √	——	dt. c. q.  (3)
	dx

That is to say, if you wish to deal with the relation of heat, chemical action and light working in the same phenomenological field at the same time, you have to use imaginary numbers — your calculation has to involve the mathematical relations expressed in imaginary numbers. But now we have already made the following statement. The spectral band that we can produce experimentally under terrestrial conditions is to be thought of actually as a circle that has been opened out. Furthermore, the complete spectrum has the peach blossom color above. If, by the employment of a sufficiently great force, you were able to bend the spectrum into a circle, you would bring together what apparently extends off into infinity in either direction. Now you can realize that this closing up cannot simply be thought of as being carried out in a circle in one plane. For as you go out into the heat region you also go off to one side (i.e. into something qualitatively different) and, proceeding into the chemical effect region, you go off to the other side. You are then in a situation where you must go first into the infinite on one side and then into the infinite on the other side and then into the infinite on the other side. You have first the awkward problem of going into infinity in a plane in one direction and then coming back from infinity and entering the plane on the other side. This implies that you reach the same infinite point no matter what direction you take. Moreover, you are confused unless you assume that you reach the same point as you go out in one direction and then in the other and you then have to come back from two different points at infinity. The way to discovery of the peach blossom color is thus a doubly complicated one. Not only must you bend the spectrum in one plane, but at right angles with, say an electromagnet, you will have to turn the magnet. That, however, lead to another point. If the magnet would have to be turned, then none of the mathematical expressions so far given would apply entirely. We then have to call in what was put before you yesterday in the discussion following the lecture by Messrs. Blumel and Strakesch, namely the super-imaginary number. You will doubtless recollect that we have to take into account that there is controversy about these super-imaginary numbers. They are readily handled mathematically and have, so to speak, more than one meaning. Some mathematicians even question whether there is any justification for them at all. Physics does not give us a definite formulation of the super-imaginary numbers. Nevertheless we put them into the series because we are led to see that they are necessary if we wish to formulate in an orderly manner what happens in the realm of chemical activity, light, heat, and what takes place in addition when we pass out in one direction through this series and come back into it from the other direction.

One who has the organ to perceive these things finds something very peculiar. He finds something which, I believe, furnishes a real foundation for illuminating the basic facts of physical phenomena. What I mean my friends, is this. The same sort of difficulty that meets one in the consideration of super-imaginary numbers also meets one when the attempt is made to apply the science of the inorganic to the phenomena of life. It cannot be done with these concepts of the inorganic. They simply do not apply. What has been the result of this? On the one hand there are thinkers who say: “The organic things of the earth have arisen by a transformation out of the inorganic.” But with this view alone one can never enter the reality of the living. Other thinkers like Prayer, regard the organic as the source of the inorganic and come nearer the truth. They think of the earth as originally a living body and what is today inorganic they consider as something thrown off or as that which has died out of the organic. But these people do not make us an entirely satisfactory picture.

The same difficulty that meets us in the phenomena of nature considered by and for themselves is met also when we attempt a comprehensive formulation of what is present in the realms of heat, light, and chemical activity and what is come upon when we attempt to close the color band in a natural manner. We must assume, of course, that this color band can be closed somewhere although it is obvious that it cannot be done under terrestrial conditions.

It is necessary for us to recognize how the purely mathematical leads up to the problem of living. With the faculties at hand today you can handle the phenomena of light, heat and chemical action, let us say, but you cannot handle what is evidently connected with these, namely the opening up of the spectrum. This cannot be formulated in a manner corresponding to the others.

It will be helpful to us at this stage if we set up a terminology. We can base this terminology on rather definite concepts. We say: Something real is at the basis of the formula for W. Let us speak of this as heat ether. Likewise something real is involved when we change the positive signs of the heat formula to negative ones, and here we speak of the chemical ether. Where our formulae involve imaginary numbers, we speak of the light ether. You see here an interesting parallelism between thinking in mathematics and thinking within science itself. The parallelism shows how we are really dealing not so much with an objective difficulty but rather with a subjective one. For the purely mathematical difficulty arises of itself, and independently of the science of external things. No one would think that a beautifully built lecture could be delivered on the limits of mathematical thinking, similar to the one du Bois-Reymond delivered on the limits of knowledge of nature. At least the conclusions would be different. Within mathematics, unless the matter slips us because it is too complicated, in this realm of the purely mathematical it must be possible to set up a completely formulated expression. The fact that one cannot do this hangs together with our own relative lack of maturity. It is unthinkable that we have here an absolute shortcoming or limit to human knowledge. It is extremely important that you hold this before your minds as a fundamental. For this shows us how we cannot apply mathematics if we wish to enter reality unless we keep in mind certain relations. We cannot simply say with the energeticists, for instance, “a given quantity of heat changes into a certain quantity of chemical energy and vice versa.” That we cannot do, but we must bring in certain other values when a process of this kind takes place. For the necessity of the case constrains us to see as essential not the quantitative mechanical change from one energy to another but rather the qualitative aspect of the transformation. This is indeed to be found along with the quantitative.

If people turned their attention to these qualitative changes which are expressed by the numerical formulations, such ideas as the following would not be advanced: “Apparently heat is just heat because we experience it as such, mechanical energy is as we experience it, chemical energy is what we see as chemical processes; but within, these processes are all alike. Mechanical energy is manifesting everywhere and heat is nothing but a form of this energy.”

This idea of a bombardment, of collisions between molecules and atoms or between these and the wall of the vessel — this struggle for an abstract unity of all energy which makes it into a mechanical motion and nothing more — such things as these would not have arisen if it had been seen that even when we calculate we must take into account the qualitative differences between various forms of energy. It is very interesting in this connection to see how Eduard von Hartmann was obliged to find definitions for physics that excluded the qualitative. Naturally, one cannot find this in the one-sided mathematics of physics, and aside from the cases where negative quantities arise from purely mathematical relations, physicists do not like to reckon with numerical quality differences. They use positive and negative signs, but only because of purely mathematical relationships. In the ordinary theory of energy, justification would never be found for making one energy positive and another negative on the basis of qualitative differences.

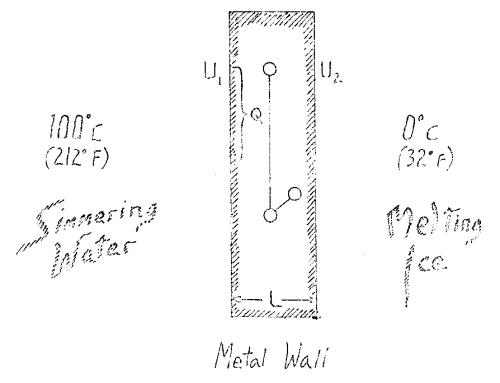
Figure 1

Figure 2