|
Lecture III
Dornach,
December 26, 1922
In the
last two lectures I tried to indicate the point in time when the
scientific outlook and manner of thinking, such as we know it today,
arose in the course of time. It was pointed out yesterday that the
whole character of this scientific thinking, emerging at the
beginning most clearly in Copernicus’ conception of astronomy,
depends on the way in which mathematical thinking was gradually
related to the reality of the external world. The development of
science in modern times has been greatly affected by a change —
one might almost say a revolutionary change — in human
perception in regard to mathematical thinking itself. We are much
inclined nowadays to ascribe permanent and absolute validity to our
own manner of thinking.
Nobody notices how much matters have changed. We take a certain
position today in regard to mathematics and to the relationship of
mathematics to reality. We assume that this is the way it has to be
and that this is the correct relationship. There are debates about it
from time to time, but within certain limits this is regarded as the
true relationship. We forget that in a none too distant past mankind
felt differently concerning mathematics. We need only recall what
happened soon after the point in time that I characterized as the
most important in modern spiritual life, the point when Nicholas
Cusanus presented his dissertation to the world. Shortly after this,
not only did Copernicus try to explain the movements of the solar
system with mathematically oriented thinking of the kind to which we
are accustomed today, but philosophers such as Descartes and Spinoza
[ 24 ]
began to apply this mathematical thought to the whole physical and
spiritual universe.
Even in such a book as his Ethics, the philosopher Spinoza
placed great value on presenting his philosophical principles and
postulates, if not in mathematical formulae — for actual
calculations play no special part — yet in such a manner that
the whole form of drawing conclusions, of deducing the later rules
from earlier ones, is based on the mathematical pattern. By and by it
appeared self-evident to the men of that time that in mathematics
they had the right model for the attainment of inward certainty.
Hence they felt that if they could express the world in thoughts
arranged in the same clear-cut architectural order as in a
mathematical or geometrical system, they would thereby achieve
something that would have to correspond to reality. If the character
of scientific thinking is to be correctly understood, it must be
through the special way in which man relates to mathematics and
mathematics relates to reality. Mathematics had gradually become what
I would term a self-sufficient inward capacity for thinking. What do
I mean by that?
The mathematics existing in the age of Descartes
[ 25 ]
and Copernicus can certainly be described more or less in the same terms
as apply today. Take a modern mathematician, for example, who teaches
geometry, and who uses his analytical formulas and geometrical concepts
in order to comprehend some physical process. As a geometrician, this
mathematician starts from the concepts of Euclidean geometry, the
three-dimensional space (or merely dimensional space, if he thinks of
non-Euclidean geometry.)
[ 26 ]
In three-dimensional space he distinguishes
three mutually perpendicular directions that are otherwise identical.
Space, I would say, is a self-sufficient form that is simply placed
before one's consciousness in the manner described above
without questions being raised such as: Where does this form come
from? Or, Where do we get our whole geometrical system?
In view of the increasing superficiality of psychological thinking,
it was only natural that man could no longer penetrate to those inner
depths of soul where geometrical thought has its base. Man takes his
ordinary consciousness for granted and fills this consciousness with
mathematics that has been thought-out but not experienced. As an
example of what is thought-out but not experienced, let us consider
the three perpendicular dimensions of Euclidean space. Man would have
never thought of these if he had not experienced a threefold
orientation within himself. One orientation that man experiences in
himself is from front to back. We need only recall how, from the
external modern anatomical and physiological point of view, the
intake and excretion of food, as well as other processes in the human
organism, take place from front to back. The orientation of these
specific processes differs from the one that prevails when, for
example, I do something with my right arm and make a corresponding
move with my left arm. Here, the processes are oriented left and
right. Finally, in regard to the last orientation, man grows into it
during earthly life. In the beginning he crawls on all fours and only
gradually, stands upright, so that this last orientation flows within
him from above downward and up from below.
As matters stand today, these three orientations in man are regarded
very superficially. These processes — front to back, right to
left or left to right, and above to below — are not inwardly
experienced so much as viewed from outside. If it were possible to go
back into earlier ages with true psychological insight, one would
perceive that these three orientations were inward experiences for
the men of that time. Today our thoughts and feelings are still
halfway acknowledged as inward experiences, but he man of a bygone
age had a real inner experience, for example, of the front-to-back
orientation. He had not yet lost awareness of the decrease in
intensity of taste sensations from front to back in the oral cavity.
The qualitative experience that taste was strong on the tip of the
tongue, then grew fainter and fainter as it receded from front to
back, until it disappeared entirely, was once a real and concrete
experience. The orientation from front to back was felt in such
qualitative experiences. Our inner life is no longer as intense as it
once was. Therefore, today, we no longer have experiences such as
this. Likewise man today no longer has a vivid feeling for the
alignment of his axis of vision in order to focus on a given point by
shifting the right axis over the left. Nor does he have a full
concrete awareness of what happens when, in the orientation of
right-left, he relates his right arm and hand to the left arm and
hand. Even less does he have a feeling that would enable him to say:
The thought illuminates my head and, moving in the direction from
above to below, it strikes into my heart. Such a feeling, such an
experience, has been lost to man along with the loss of all
inwardness of world experience. But it did once exist. Man did once
experience the three perpendicular orientation of space within
himself. And these three spatial orientations — right-left,
front-back, and above-below — are the basis of the
three-dimensional framework of space, which is only the abstraction
of the immediate inner experience described above.
So what can we say when we look back at the geometry of earlier
times? We can put it like this: It was obvious to a man in those ages
that merely because of his being human the geometrical elements
revealed themselves in his own life. By extending his own
above-below, right-left, and front-back orientations, he grasped the
world out of his own being.
Try to sense the tremendous difference between this mathematical
feeling bound to human experience, and the bare, bleak mathematical
space layout of analytical geometry, which establishes a point
somewhere in abstract space, draws three coordinating axes at right
angles to each other and thus isolates this thought-out space scheme
from all living experience. But man has in fact torn this thought-out
spatial diagram out of his own inner life. So, if we are to
understand the origin of the later mathematical way of thinking that
was taken over by science, if we are to correctly comprehend its
self-sufficient presentation of structures, we must trace it back to
the self-experienced mathematics of a bygone age. Mathematics in
former times was something completely different. What was once
present in a sort of dream-like experience of three-dimensionality
and then became abstracted, exists today completely in the
unconscious. As a matter of fact, man even now produced mathematics
from his own three-dimensionality. But the way in which he derives
this outline of space from his experiences of inward orientation is
completely unconscious. None of this rises into consciousness except
the finished spatial diagram. The same is true of all completed
mathematical structures. They have all been severed from their roots.
I chose the example of the space scheme, but I could just as well
mention any other mathematical category taken from algebra or
arithmetic. They are nothing but schemata drawn from immediate human
experience and raised into abstraction.
Going back a few centuries, perhaps to the fourteenth century, and
observing how people conceived of things mathematical, we find that
in regard to numbers they still had an echo of inward feelings. In an
age in which numbers had already become an abstract ads they are
today, people would have been unable to find the names for numbers.
The words designating numbers are often wonderfully characteristic.
Just think of the word “two.” (zwei) It clearly expresses
a real process, as when we say entzweien, “to cleave in
twain.” Even more, it is related to zweifeln, “to
doubt.” It is not mere imitation of an external process when
the number two, zwei, is described by the word
Entzweien,
which indicates the disuniting, the splitting, of something formerly
a whole. It is in fact something that is inwardly experienced and
only then made into a scheme. It is brought up from within, just as
the abstract three-dimensional space-scheme is drawn up from inside
the mind.
We arrive back at an age of rich spiritual vitality that still
existed in the first centuries of Christianity, as can be
demonstrated by the fact that mathematics, mathesis, and mysticism
were considered to be almost one and the same. Mysticism, mathesis,
and mathematics are one, though only in a certain connection. For a
mystic of the first Christian centuries, mysticism was something that
one experienced more inwardly in the soul. Mathematics was the
mysticism that one experienced more outwardly with the body; for
example, geometry with the body's orientations to
front-and-back, right-and-left, and up-and-down. One could say that
actual mysticism was soul mysticism and that mathematics, mathesis,
was mysticism of the corporeality. Hence, proper mysticism was
inwardly experienced in what is generally understood by this term;
whereas mathesis, the other mysticism, as experienced by means of an
inner experience of the body, as yet not lost.
As a matter of fact, in regard to mathematics and the mathematical
method Descartes and Spinoza still had completely different feelings
from what we have today. Immerse yourself in these thinkers, not
superficially as in the practice today when one always wants to
discover in the thinkers of old the modern concepts that have been
drilled into our heads, but unselfishly, putting yourself mentally in
their place. You will find that even Spinoza still retained something
of a mystical attitude toward the mathematical method.
The philosophy of Spinoza differs from mysticism only in one respect.
A mystic like Meister Eckhart or Johannes Tauler
[ 27 ]
attempts to experience the cosmic secrets more in the depths of feeling.
Equally inwardly, Spinoza constructs the mysteries of the universe along
mathematical, methodical lines, not specifically geometrical lines,
but lines experienced mentally by mathematical methods. In regard to
soul configuration and mood, there is no basic difference between the
experience of Meister Eckhart's mystical method and
Spinoza's
mathematical one. Anyone how makes such a distinction does not really
understand how Spinoza experienced his Ethics, for example, in a
truly mathematical-mystical way. His philosophy still reflects the
time when mathematics, mathesis, and mysticism were felt as one and
the same experience in the soul.
Now, you will perhaps recall how, in my book
The Case for Anthroposophy,
[ 28 ]
I tried to explain the human organization in a way
corresponding to modern thinking. I divided the human organization
—
meaning the physical one — into the nerve-sense system, the
rhythmic system, and the metabolic-limb system. I need not point out
to you that I did not divide man into separate members placed side by
side in space, although certain academic persons have accused
[ 29 ]
me of such a caricature. I made it clear that these three systems
interpenetrate each other. The nerve-sense system is called the
“head
system” because it is centered mainly in the head, but it
spreads out into the whole body. The breathing and blood rhythms of
the chest system naturally extend into the head organization, and so
on. The division is functional, not local. An inward grasp of this
threefold membering will give you true insight into the human being.
Let us now focus on this division for a certain purpose. To begin
with, let us look at the third member of the human organization, that
of digestion (metabolism) and the limbs. Concentrating on the most
striking aspect of this member, we see that man accomplishes the
activities of external life by connecting his limbs with his inner
experiences. I have characterized some of these, particularly the
inward orientation experience of the three directions of space. In
his external movements, in finding his orientation in the world,
man's limb system achieves inward orientation in the three
directions. In walking, we place ourselves in a certain manner into
the experience of above-below. In much that we do with our hands or
arms, we bring ourselves into the orientation of right-and-left. To
the extent that speech is a movement of the aeriform in man, we even
fit ourselves into direction of front-and-back, back-and-front, when
we speak. Hence, in moving about in the world, we place our inward
orientation into the outer world.
Let us look at the true process, rather than the merely illusionary
one, in a specific mathematical case. It is an illusionary process,
taking place purely in abstract schemes of thought, when I find
somewhere in the universe a process in space, and I approach it as an
analytical mathematician in such a way that I draw or imagine the
three coordinate axes of the usual spatial system and arrange this
external process into Descartes’ purely artificial space
scheme.

This is what occurs above, in the realm of thought schemes, through
the nerve-sense system. One would not achieve a relationship to such
a process in space if it were not for what one does with one's
limbs, with one's whole body, if it were not for inserting
oneself into the whole world in accordance with the inward
orientation of above-below, right-left, and front-back. When I walk
forward, I know that on one hand I place myself in the vertical
direction in order to remain upright. I am also aware that in walking
I adjust my direction to the back-to-front orientation, and when I
swim and use my arms, I orient myself in right and left. I do not
understand all this if I apply Descartes’ space scheme, the
abstract scheme of the coordinate axes. What gives me the impression
of reality in dealing with matters of space is found only when I say
to myself: Up in the head, in the nerve system, an illusory image
arises of something that occurs deep down in the subconscious. Here,
where man cannot reach with his ordinary consciousness, something
takes place between his limb system and the universe. The whole of
mathematics, of geometry, is brought up out of our limb system of
movement. We would not have geometry if we did not place ourselves
into the world according to inward orientation. In truth, we
geometrize when we lift what occurs in the subconscious into the
illusory of the thought scheme. This is the reason why it appears so
abstractly independent to us. But his is something that this only
come about in recent times. In the age in which mathesis,
mathematics, was still felt to be something close to mysticism, the
mathematical relationship to all things was also viewed as something
human.
Where is the human factor if I imagine an abstract point somewhere in
space crossed by three perpendicular directions and then apply this
scheme to a process perceived in actual space? It is completely
divorced from man, something quite inhuman. This non-human element,
which has appeared in recent times in mathematical thinking, was once
human. But when was it human?
The actual date has already been indicated, but the inner aspect is
still to be described. When was it human? It was human when man did
not only experience in his movements and his inward orientation in
space that he stepped forward from behind and moved in such a way
that he was aware of his vertical as well as the horizontal
direction, but when he also felt the blood's inward activity in
all such moving about, in all such inner geometry. There is always
blood activity when I move forward. Think of the blood activity
present when, as an infant, I lifted myself up from the horizontal to
an upright position! Behind man's movements, behind his
experience of the world by virtue of movements, (which can also be,
and at one time was, an inward experience) there stands the
experience of the blood. Every movement, small or large, that I
experience as I perform it contains its corresponding blood
experience. Today blood is to us the red fluid that seeps out when we
prick our skin. We can also convince ourselves intellectually of its
existence. But in the age when mathematics, mathesis, was still
connected with mysticism, when in a dreamy way the experience of
movement was inwardly connected with that of blood, man was inwardly
aware of the blood. It was one thing to follow the flow of blood
through the lungs and quite another to follow it through the head.
Man followed the flow of the blood in lifting his knee or his foot,
and he inwardly felt and experienced himself through and through in
his blood. The blood has one tinge when I raise my foot, another when
I place it firmly on the ground. When I lounge around and doze
lazily, the blood's nuance differs from the one it has when I
let thoughts shoot through my head. The whole person can take on a
different form when, in addition to the experience of movement, he
has that of the blood. Try to picture vividly what I mean. Imagine
that you are walking slowly, one step at a time; you begin to walk
faster; you start to run, to turn yourself, to dance around. Suppose
that you were doing all this, not with today's abstract
consciousness, but with inward awareness: You would have a different
blood experience at each stage, with the slow walking, then the
increase in speed, the running, the turning, the dancing. A different
nuance would be noted in each case. If you tried to draw this inner
experience of movement, you would perhaps have to sketch it like this
(white line.) But for each position in which you found yourself
during this experience of movement, you would draw a corresponding
inward blood experience (red, blue, yellow — see Figure 2)
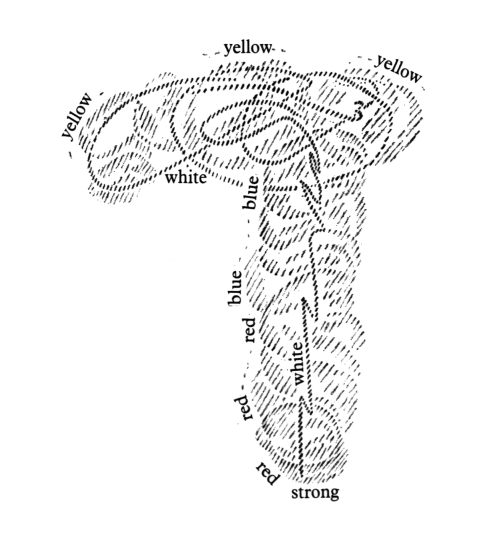
Of the first experience, that of movement, you would say that you
have it in common with external space, because you are constantly
changing your position. The second experience, which I have marked by
means of the different colors, is a time experience, a sequence of
inner intense experiences.
In fact, if you run in a triangle, you can have one inner experience
of the blood. You will have a different one if you run in a
square.
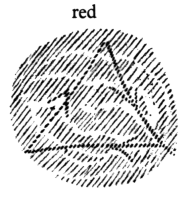
What is outwardly quantitative and geometric, is inwardly intensely
qualitative in the experience of the blood.
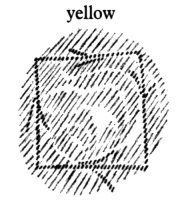
It is surprising, very surprising, to discover that ancient
mathematics spoke quite differently about the triangle and the
square. Modern nebulous mystics describe great mysteries, but there
is no great mystery here. It is only what a person would have
experienced inwardly in the blood when he walked the outline of a
triangle or a square, not to mention the blood experience
corresponding to the pentagram. In the blood the whole of geometry
becomes qualitative inward experience. We arrive back at a time when
one could truly say, as Mephistopheles does in Goethe's
Faust,
“Blood is a very special fluid.”
[ 30 ]
This is because, inwardly experienced, the blood absorbs all geometrical
forms and makes of them intense inner experiences. Thereby man learns to
know himself as well. He learns to know what it means to experience a
triangle, a square, a pentagram; he becomes acquainted with the
projection of geometry on the blood and its experiences. This was
once mysticism. Not only was mathematics, mathesis, closely related
to mysticism, it was in fact the external side of movement, of the
limbs, while the inward side was the blood experience. For the mystic
of bygone times all of mathematics transformed itself out of a sum of
spatial formations into what is experienced in the blood, into an
intensely mystical rhythmic inner experience.
We can say that once upon a time man possessed a knowledge that he
experienced, that he was an integral part of; and that at the point
in time that I have mentioned, he lost this oneness of self with the
world, this participation in the cosmic mysteries. He tore
mathematics loose from his inner being. No longer did he have the
experience of movement; instead, he mathematically constructed the
relationships of movement outside. He no longer had the blood
experience; the blood and its rhythm became something quite foreign
to him. Imagine what this implies: Man tears mathematics free from
his body and it becomes something abstract. He loses his
understanding of the blood experience. Mathematics no longer goes
inward. Picture this as a soul mood that arose at a specific time.
Earlier, the soul had a different mood than later. Formerly, it
sought the connection between blood experience and experience of
movement; later, it completely separated them. It no longer related
the mathematical and geometrical experience to its own movement. It
lost the blood experience. Think of this as real history, as
something that occurs in the changing moods of evolution. Verily, a
man who lived in the earlier age, when mathesis was still mysticism,
put his whole soul into the universe. He measured the cosmos against
himself. He lived in astronomy.
Modern man inserts his system of coordinates into the universe and
keeps himself out of it. Earlier, man sensed a blood experience with
each geometrical figure. Modern man feels no blood experience; he
loses the relationship to his own heart, where the blood experiences
are centered. Is it imaginable that in the seventh or eighth century,
when the soul still felt movement as a mathematical experience and
blood as a mystical experience, anybody would have founded a
Copernican astronomy with a system of coordinates simply inserted
into the universe and totally divorced from man? No, this became
possible only when a specific soul constitution arose in evolution.
And after that something else became possible as well. The inward
blood awareness was lost. Now the time had come to discover the
movements of the blood externally through physiology and anatomy.
Hence you have this change in evolution: On one hand Copernican
astronomy, on the other the discovery of the circulation of the blood
by Harvey,
[ 31 ]
a contemporary of Bacon and Hobbes. A world view gained by
abstract mathematics cannot produce anything like the ancient
Ptolemaic theory, which was essentially bound up with man and the
living mathematics he experienced within himself. Now, one
experiences an abstract system of coordinates starting with an
arbitrary zero point. No longer do we have the inward blood
experience; instead, we discover the physical circulation of the
blood with the heart in the center.
The birth of science thus placed itself into the whole context of
evolution in both its conscious and unconscious processes. Only in
this way, out of the truly human element, can one understand what
actually happened, what had to happen in recent times for science
—
so self-evident today — to come into being in the first place.
Only thus could it even occur to anybody to conduct such
investigations as led, for example, to Harvey's discovery of
the circulation of the blood. We shall continue with this
tomorrow.
|